I've enjoyed straightedge-and-compass constructions since high school—I remember that high school geometry was my favorite math class up until to a point in college after I had already decided to be a math major. And I also remember feeling cheated in high school geometry because our textbook used a construction system based on folding paper instead of a straightedge and compass!
Lately I've been painting a lot, and what I've been painting is the constructions in Book 1 of Euclid's Elements, a weirdly enduring piece of the mathematics canon. Written around 300 BCE, I think this is the oldest piece of mathematical writing I read any substantial amount of during my mathematics education by around 2000 years! (Most people could probably add another ~100-300 years on there... but at one point I assisted a professor with a project of translating an early calculus textbook from French to English.)
 |
| Gouache paintings of all 14 constructions from Book 1 |
All the paintings here use gouache on 5x7 Yupo heavy watercolor paper. This blog does pretty badly with photos; they might look nicer on instagram, where I'm planning to post one construction at a time. They look best in person, though, and I would happily show them off to you.
Individual Constructions
Numbering below follows the original: in Elements the constructions are interspersed with other propositions.
1. Equilateral Triangle
I find this surprising as a first proposition... the construction is so simple, and recurs so many times throughout what follows, that it's obvious in a practical sense why Euclid starts here. But my intuition insists that a line or an angle is a more fundamental building block of geometry than an equilateral triangle.
2. A straight line of given length
Another construction that will recur frequently.
I wasn't satisfied with how close in hue the given point and line here are to the constructed ones. In later paintings I tried to create more separation to make the construction easier to follow.
3. Cutting a given length from a given line
This construction is almost identical to the previous one; only one more circle is constructed.
9. Angle bisection
Between constructions 3 and 9 Euclid proves several propositions relating mainly to congruent triangles. These are used in verifying that the next set of constructions are correct.
This is one of my favorite paintings from the series; I loved the way the darker blue paint behaved.
10. Segment bisection
Another surprise for my intuition... intuitively, I find it much easier to approximate bisecting a line segment than an angle. But this construction relies on the previous one.
11. A perpendicular to a line from a point on the line
The second of three very similar constructions.
This painting bothers me... something about the eye I can see in it.
12. A perpendicular to a line from a point not on the line
The triangles here are not needed for the construction, but for the proof that the lines really are perpendicular.
22. Given three appropriate lines, to construct a triangle
This construction was so much more complex than the preceding ones that it broke parts of the system I'd been using.
The first few times I tried it, I kept losing track of which lines, points, and circles were which; I started color coding as I went instead of, as I had been doing, at the end. Then I started omitting some of the full circles used in the construction for transferring lengths, instead making just the hatches where they intersect (and only the intersection that is used moving forward). This simplification also made the appearance a lot nicer, so I kept it for the final painting; it's also used in some of the other constructions that follow.
This was also the only construction for which I didn't fit Euclid's full specifications on the page. The whole statement is: "To construct a triangle out of three straight lines which equal three given straight lines: thus it is necessary that the sum of any two of the straight lines should be greater than the remaining one."
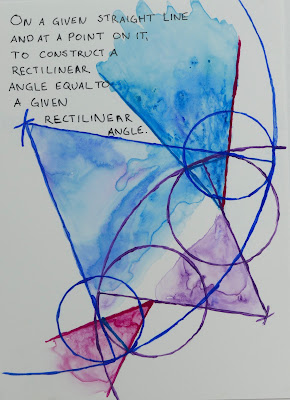
23. To copy an angle at a given point on a line
This is really another construction of a triangle, this time in a specific place... but since you can choose two sides to be the same length, it is a little less complicated, both to carry out and to look at.
31. A parallel line running through a given point
This is the first construction after Euclid starts using the "
parallel postulate." If you'd like to explore what might happen without that postulate, one place you can start is this
spherical geometry model: spherical geometry shares the first four of Euclid's postulates, but instead of
some lines intersecting as specified in the parallel postulate,
any two lines intersect.
42. A parallelogram with area equal to that of a triangle, on a given angle
Euclid states a number of theorems as pertaining to parallelograms where, practically, one would most likely want to use the special case parallelogram of a rectangle. If I'd painted a rectangle, though, I'd expect people to feel unsure whether the same construction works for other parallelograms. A rectangle just feels very special.
This is another construction that was a breaking point for my system. Up to this point, I'd used the method from construction 2 every time I needed to transfer a length. With a typical modern compass, this is not necessary—it stays fixed at the same length until it is adjusted. I found this construction unbearably messy, both to do and to look at afterwards, when performed using all the steps from construction 2. So I started using my compass to transfer lengths in a single step. I didn't want to—I did drafts both on paper and with
geometry software that let me play with the arrangement more efficiently, trying to find a position or choice of angles that would clean things up. I couldn't do it, though.
44. A parallelogram with area equal to a triangle, on a given line and angle
This construction allows you to specify the length of one side of the parallelogram constructed. For example, you could use a side one unit long. Or, as in the next construction...
45. A parallelogram equal in area to a polygon
... you could chain multiple parallelogram constructions together, to construct a parallelogram with the same area as a more complicated shape. Here I've used a quadrilateral for simplicity, but any shape with straight sides would work, as it can be divided into triangles and then construction 44 can be applied to each in sequence.
Here I've used a combination of transferring lengths with my compass and only marking portions of some circles. By this point in the book there are also multiple ways to perform the constructions: for example, I've used a different method for constructing the parallel lines around the original figure here than in construction 44.
46. A square
A return to simplicity... this was a relief after the last several parallelogram constructions of increasing complexity. It was nice to have relatively large areas to play with paint in again.